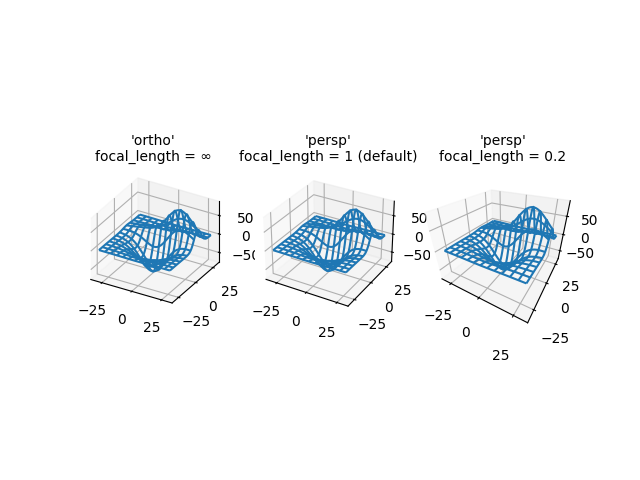
3D 图投影类型

演示了 3D 图的不同相机投影，以及更改透视投影的焦距的影响。请注意，Matplotlib 会修正更改焦距引起的“缩放”效果。

默认焦距为 1，对应于 90 度的视野 (FOV)。焦距在 1 和无穷大之间增加会“平坦化”图像，而焦距在 1 和 0 之间减小会夸大透视，使图像具有更明显的深度。在极限情况下，焦距为无穷大对应于在修正缩放效果后进行正射投影。

您可以通过以下公式从 FOV 计算焦距

\[1 / \tan (\mathrm{FOV} / 2)\]

或反之

\[\mathrm{FOV} = 2 \arctan (1 / \mathrm{focal length})\]

import matplotlib.pyplot as plt

from mpl_toolkits.mplot3d import axes3d

fig, axs = plt.subplots(1, 3, subplot_kw={'projection': '3d'})

# Get the test data
X, Y, Z = axes3d.get_test_data(0.05)

# Plot the data
for ax in axs:
    ax.plot_wireframe(X, Y, Z, rstride=10, cstride=10)

# Set the orthographic projection.
axs[0].set_proj_type('ortho')  # FOV = 0 deg
axs[0].set_title("'ortho'\nfocal_length = ∞", fontsize=10)

# Set the perspective projections
axs[1].set_proj_type('persp')  # FOV = 90 deg
axs[1].set_title("'persp'\nfocal_length = 1 (default)", fontsize=10)

axs[2].set_proj_type('persp', focal_length=0.2)  # FOV = 157.4 deg
axs[2].set_title("'persp'\nfocal_length = 0.2", fontsize=10)

plt.show()